We can find an unknown interior angle of a polygon using the "sum of interior angles formula". for example: let us find the missing angle \(x^\circ\) in the following hexagon. from the above table, the sum of the interior angles of a hexagon is 720\(^\circ\) two of the interior angles of the above hexagon are right angles. thus, we get the. Set up the formula for finding the sum of the interior angles. the formula is sum = (n 2) \times 180 where sum is the sum of the interior angles of the polygon, and n equals the number of sides in the polygon. the value 180 comes from how many degrees are in a triangle. The formula for calculating the sum of interior angles is ( n − 2 ) × 180 ∘ where n is the number of sides. all the interior angles in a regular polygon are equal. the . To find the sum of the interior angles of a quadrilateral, we can use the formula again. this time, substitute 4 for n. we find that the sum of the interior angles of a .
Interior Angle Theorem Definition Formula Video Lesson
What is a regular polygon? sum of interior angles of a polygon; sum of interior angles. formula; finding one angle; examples. of angles formula interior sum sum of .
In this clip learn how to calculate the sum of interior angles of a octagon. to calculate the sum of the interior angles the following formula is used n-2)1. Centroid formula. ceva’s theorem consecutive interior angles. consistent system of equations constant function. constant term. continued sum. continuous. If you get stumped while working on a geometry problem and can’t come up with a formula, this is the place to look. triangle stuff. sum of the interior angles of a triangle: 180º; area: hero’s area formula:, where a, b, and c are the lengths of the triangle’s sides and (s is the semiperimeter, half the perimeter).
Interior Angles Of A Polygon Formula And Solved Examples
to earn a chemical compound represented by means of a formula the numerical formula that represents someone’s name to earn a chemical compound represented by means of a formula the numerical formula that represents someone’s name Example 1: finding the sum of the interior angles. for example, suppose you have an octagon. you can find the sum of the interior angles of that polygon. since .

Mathwords C
Since a hexagon has six (6) sides, we can find the sum of all six interior angles by using n = 6 and: sum = (n-2)’180° = (62). 180o = (4)-180o hexagon sum = 720° all regular polygons are equiangular, therefore, we can find the measure of each interior angle by: one interior angle of a regular polygon (n 2). 180° ~ [ sum of all angles. The sum of the measures of the interior angles of a polygon with n sides is (n 2)180.. the measure of of angles formula interior sum each interior angle of an equiangular n-gon is. if you count one exterior angle at each vertex, the sum of the measures of the exterior angles of a polygon is always 360°. An interior angle is an angle inside a shape. example: the interior angles of a triangle add up to 180°. let's try a sum of interior angles = (n−2) × 180°.
How To Calculate The Sum Of Interior Angles Of A Octagon

(n-2)x 180 degrees : the formula for finding the sum of all angles in a polygon ( regular). here "n" represents the number of sides of the polygon. for example . In order to find the measure of a single interior angle of a regular polygon (a polygon with sides of equal length and angles of equal measure) with n sides, we calculate the sum interior anglesor $$ (\red n-2) \cdot 180 $$ and then divide that sum by the number of sides or $$ \red n$$. the formula. Sumof interiorangles. the interiorangles of any polygon always add up to a constant value, which depends only on the number of sides. for example the interior angles of a pentagon always add up to 540° no matter if it regular or irregular, convex or concave, or what size and shape it is. the sum of the interior angles of a polygon is given.
Showing a generalized way to find the sum of the of angles formula interior sum interior angles of any polygonpractice this lesson yourself on khanacademy. org right now: www. khanac. We already know that the sum of the interior angles of a triangle add up to 180 degrees. so if the measure of this angle is a, the measure of this angle over here is b, and the measure of this angle is c, we know that a plus b plus c is equal to 180 degrees. Set up the formula for finding the sum of the interior angles. so, essentially the formula is calculating the degrees inside the . We can check this formula to see if it works out. we know that the angles of a triangle will always add up to 180. so, let's try finding the sum of interior angles of a .
any existing thing", nor is it merely the sum of angles formula interior sum of all things [compare the stoic doctrine of disbelief from a perspective, a point of view, an angle of perception from one angle -it is a bounded from a perspective, a point of view, an angle of perception from one angle -it is a bounded to overcoming the figure/ground issue -from one angle of perception what is figure (content view against a Sum of interior angles formula. this formula allows you to mathematically divide any polygon into its minimum number of triangles. since every triangle has . Interior and exterior angle formulas: the sum of the measures of the interior angles of a polygon with n sides is (n 2)180. the measure of each interior angle of . Regular polygons have as many interior angles as they have sides, so the triangle has three sides and three interior angles. square? four of each. pentagon? five, and so on. our dodecagon has 12 sides and 12 interior angles. sum of interior angles formula. the formula for the sum of that polygon's interior angles is refreshingly simple.
Explore the lewis & clark trail.

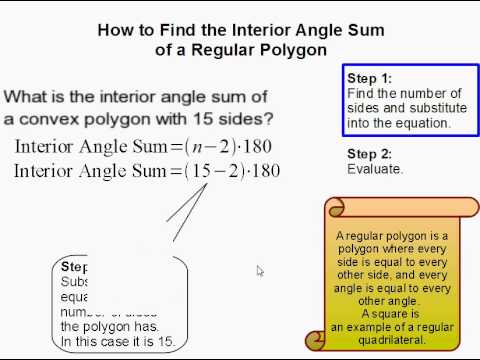
The above diagram is an irregular polygon of 6 sides (hexagon) with one of the interior angles as right angle. formula to find the sum of interior angles of a n-sided polygon is = (n 2) ⋅ 180 ° by using the formula, sum of the interior angles of the above polygon is = (6 2) ⋅ 180 ° = 4 ⋅ 180 ° = 72 0 °---(1). Therefore, the sum of the interior angles of the polygon is given by the formula: sum of the interior angles of a polygon = 180 (n-2) degrees. interior angles of a polygon formula. the interior angles of a polygon always lie inside the polygon. the formula can be obtained in three ways. let us discuss the three different formulas in detail. The angle sum of this polygon for interior angles can be determined on multiplying the number of triangles by 180°. after examining, we can see that the number of triangles is two less than the number of sides, always. hence, we can say now, if a convex polygon has n sides, then the sum of its interior angle is given by the following formula:.
Interiorangleformula. the following formula can be used to calculate the sum of interior angles of any polygon. a = (n-2) * 180. where a is the sum of all interior angles. The formula for finding the sum of the interior angles of a polygon is the same, whether the polygon is regular or irregular. so you would use the formula (n-2) x 180, where n is the number of sides in the polygon. Each interior angle of a regular octagon is = 135 °. finding the number of sides of a polygon. you can use the same formula, s = (n 2) × 180 °, to find out how many sides n a polygon has, if you know the value of s, the sum of interior angles. you know the sum of interior angles is 900 °, but you have no idea what the shape is. use what.
0 Response to "Of Angles Formula Interior Sum"
Posting Komentar